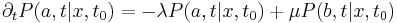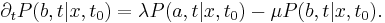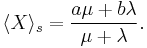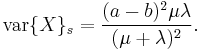Telegraph process

In probability theory, the telegraph process is a memoryless continuous-time stochastic process that shows two distinct values.

If these are called a and b, the process can be described by the following master equations:

$\partial_t P(a, t|x, t_0)=-\lambda P(a, t|x, t_0)%2B\mu P(b, t|x, t_0)$

and

$\partial_t P(b, t|x, t_0)=\lambda P(a, t|x, t_0)-\mu P(b, t|x, t_0).$

The process is also known under the names Kac process^[1] , dichotomous random process.^[2]

1 Properties
2 Application
3 See also
4 References

Properties

Knowledge of an initial state decays exponentially. Therefore for a time in the remote future, the process will reach the following stationary values, denoted by subscript s:

Mean:

$\langle X \rangle_s = \frac {a\mu%2Bb\lambda}{\mu%2B\lambda}.$

Variance:

$\operatorname{var} \{ X \}_s = \frac {(a-b)^2\mu\lambda}{(\mu%2B\lambda)^2}.$

One can also calculate a correlation function:

$\langle X(t),X(s)\rangle_s = \exp(-(\lambda%2B\mu)|t-s|) \operatorname{var} \{ X \}_s.$

Application

This random process finds wide application in model building:

In physics, spin systems and fluorescence intermittency show dichotomous properties. But especially in single molecule experiments probability distributions featuring algebraic tails are used instead of the exponential distribution implied in all formulas above.
In finance for describing stock prices^[1]

References

^ ^a ^b Bondarenko, YV (2000). "Probabilistic Model for Description of Evolution of Financial Indices". Cybernetics and systems analysis 36: 738–742. doi:10.1023/A:1009437108439.
^ Margolin, G; Barkai, E (2006). "Nonergodicity of a Time Series Obeying Lévy Statistics". Journal of Statistical Physics 122: 137–167. Bibcode 2006JSP...122..137M. doi:10.1007/s10955-005-8076-9.

Telegraph process

Contents

Properties

Application

See also

References